Annotations
Silq supports annotations !, qfree, mfree, const, and lifted.
Classical types: !
To indicate that a type τ may only hold classical values (and not
superpositions), we annotate it as $!\tau$. This ensures we can freely duplicate
and drop values of that type. For example, $1+2$ has type !int[n]. In
contrast, H(0) holds $\facs \big(\ket{0}+\ket{1} \big)$ and is thus not
classical: It has type 𝔹 and not !𝔹.
Example: The function classicalExample (below) takes two arguments x (a
classical Boolean) and f. The latter takes a classical Boolean and returns a
classical Boolean. Moreover, f itself is classical, meaning that it is
classically known.
def classicalExample(x:!𝔹,f:!𝔹!→!𝔹){
return f(x); // ^ f is classical
}
Example (not classical): The function captureQuantum (below) illustrates a
non-classical function. It returns a function captured which captures the
quantum variable x. Thus, the state of captured is in superposition, meaning
we cannot refer to captured as being classical.
def captureQuantum(x:𝔹){
captured := λ(). { // function `captured` takes no arguments
return H(x); // the body of function `captured` applies `H` to `x`
};
return captured:𝟙→𝔹;
// ^ the returned function is not classical
}
In the following, we summarize some useful properties of classical types:
- We can ignore duplicate classical annotations:
!!τ ≡ !τ - Classical commutes with tuples:
!(τ × τ) ≡ !τ × !τ(analogously for n-tuples with n>2). As a consequence, we also have!(τ × τ) ≡ !(τ × !τ) ≡ !(!τ × τ) ≡ !(!τ × !τ) - Classical commutes with arrays:
!τ[] ≡ (!τ)[] ≡ !(τ[]) - Classical commutes with fixed-length arrays:
!τ^n ≡ (!τ)^n ≡ !(τ^n) - Classical values can be re-interpreted as quantum values:
!τ <: τ
qfree
We use the annotation qfree to indicate that evaluating functions or
expressions neither introduces nor destroys superpositions. Annotation qfree
(i) ensures that evaluating qfree functions on classical arguments yields
classical results and (ii) enables automatic uncomputation.
Example 1 (not qfree): H is not qfree as it introduces superpositions: It
maps $\ket{0}$ to $\facs \Big(\ket{0}+\ket{1}\Big)$.
Example 2: X is qfreeas it neither introduces nor destroys superpositions:
It maps $\sum_{b=0}^1 \gamma_b \ket{b}$ to $\sum_{b=0}^1 \gamma_b \ket{1-b}$.
Example 3: Logical disjunction (as in x||y) is of type const 𝔹×const
𝔹→qfree 𝔹, since ORing two values neither introduces nor destroys
superpositions.
Example 4: Function myEval (below) takes a qfree function f and evaluates
it on false. Thus, myEval itself is also qfree.
def myEval(f:𝔹→qfree 𝔹)qfree{
return f(false); // ^ myEval is qfree
}
mfree
Annotation mfree indicates that a function can be evaluated without applying
any measurements.
Example: Function myEval (below) takes a mfree function as argument and
evaluates it on false. Thus, evaluate itself is also mfree.
def myEval(f:𝔹→mfree 𝔹)mfree{
return f(false); // ^ myEval is mfree
}
const
Annotation const indicates that a variable will not be changed in the given
context. Concretely, each parameter of a function and each variable in the
context may be annotated as const. We can use constant parameters and
variables more liberally, since they are guaranteed to persist in the given
context.
Example: Function myEval (below) takes a constant x and a function f that
leaves its first argument const:
def myEval(const x:𝔹,f:const 𝔹!→𝔹){
return f(x);
}
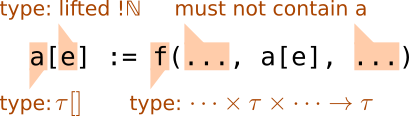
lifted
Annotation lifted is a shorthand to indicate qfree functions with only
constant arguments (classical arguments are implicitly treated as constants).
Example: Function MyOr is lifted:
def MyOr(x:𝔹, y:!𝔹)lifted{ // x and y are implicitly const
return x||y; // ^ MyOr is lifted
}